Dynamics
Dynamics calculation possibilities in FEM-Design includes footfall analysis, seismic analysis and stability analysis.
Footfall Analysis
This calculation method allows for checking a structure’s response for an exciting vibration. You can select one of the three available methods: self excitation, full excitation or rhythmic crowd load (the load case must be selected with this method).
Watch a Webinar about Footfall Analysis
Seismic Analysis
FEM-Design supports you to perform seismic analysis with the following methods of seismic dynamics calculations according to Eurocode 8.
- Modal response spectrum analysis (“Modal analysis”)
- Linear shape method (Static, linear shape)
- Mode shape method (Static, mode shape)
Seismic Loads
Seismic loads are taken into account according to the Response Spectrum Analysis method of Eurocode 8 or Turkish seismic code. Only the response spectrum and some additional parameters have to be defined as Seismic load. Required spectrums can be defined with the Seismic load by using standard spectra (automatic) or by manual definition (unique).
Results
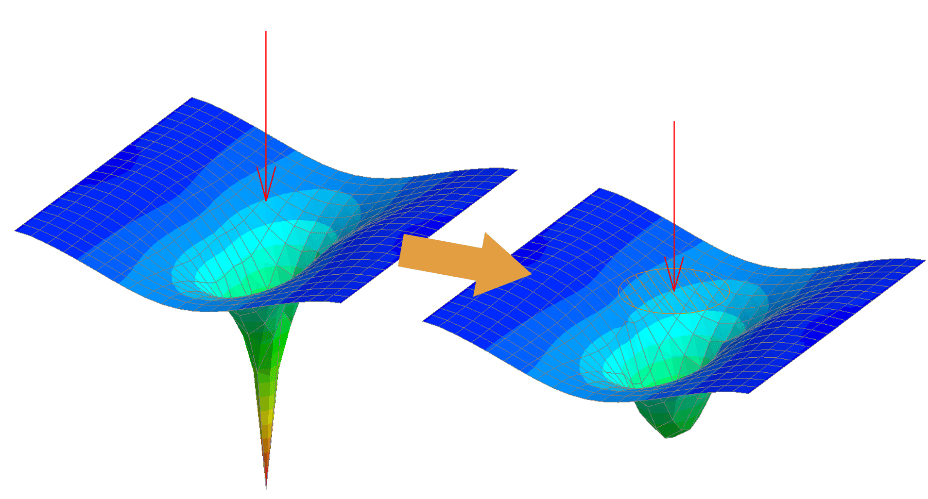
Besides displacements, reactions, connection forces, and internal forces, the program calculates the equivalent loads and the “base shear force”. Results can be displayed by vibration shape (selected at calculation settings), from torsional effect, from sums by direction and from the total sum (seismic max).
If equivalent loads are displayed, also the “base shear force” appears on screen (in grey colour). Torsional moment effect on the whole structure can also be displayed, if the torsional effect was taken into consideration during the calculation.
Watch the Seismic Analysis Overview Video
Stability Analysis
In the description of the second-order theory, it was pointed out that the resultant stiffness of the system depends on the normal force distribution. In case of linear elastic structures the geometrical stiffness matrix is a linear function of normal forces and consequently of loads:
KG (λN) = λ KG
The structure loses its loadbearing capability if the normal forces decrease the stiffness to zero, i.e. the resultant stiffness matrix becomes singular:
det [K + λ KG (N)] = 0
It is an eigenvalue calculation problem, and the smallest λ eigenvalue is the critical load parameter.
The calculation has to be performed in two steps.
First, the normal forces of the elements have to be calculated by using the K matrix.
In the second step KG and the λ parameter can be determined.
The critical load is the product of the load and the λ parameter. The above-mentioned eigenvalue problem is solved by the so-called Lanczos method in FEM-Design. The results of the calculations are as many buckling shapes as the user required and the matching λ critical load parameters.
Watch a Webinar on Stability Analysis
Why wait? Download an automated 2 x week free trial of FEM-Design and get started today!
Calculations performed according to:
Euro Code- Belgian National Annex
- British National Annex
- Danish National Annex
- Dutch National Annex
- Estonian National Annex
- Finnish National Annex
- German National Annex
Euro Code
Belgian National Annex
British National Annex
Danish National Annex
Dutch National Annex
Estonian National Annex
Finnish National Annex
German National Annex- Hungarian National Annex
- Latvian National Annex
- Norwegian National Annex
- Polish National Annex
- Romanian National Annex
- Spanish National Annex
- Swedish National Annex
- Turkish Seismic Code
Hungarian National Annex
Latvian National Annex
Norwegian National Annex
Polish National Annex
Romanian National Annex
Spanish National Annex
Swedish National Annex
Turkish Seismic CodeLanguages supported:
- English
- Finnish
- French
- Dutch
- Hungarian
- Polish
English
FrenchFEM-Design Wiki
FEM-Design allows you to perform numerous dynamics calculations: displacement, internal forces, stresses, stability, imperfections, stability analysis, eigenfrequencies and/or seismic analysis. Some extra settings such as cracked-section analysis, non-linear behaviour etc. are also available for certain modules.
Full details on all the Dynamics Analysis Types can be found on the FEM-Design Wiki by clicking here.
FEM-Design Blog
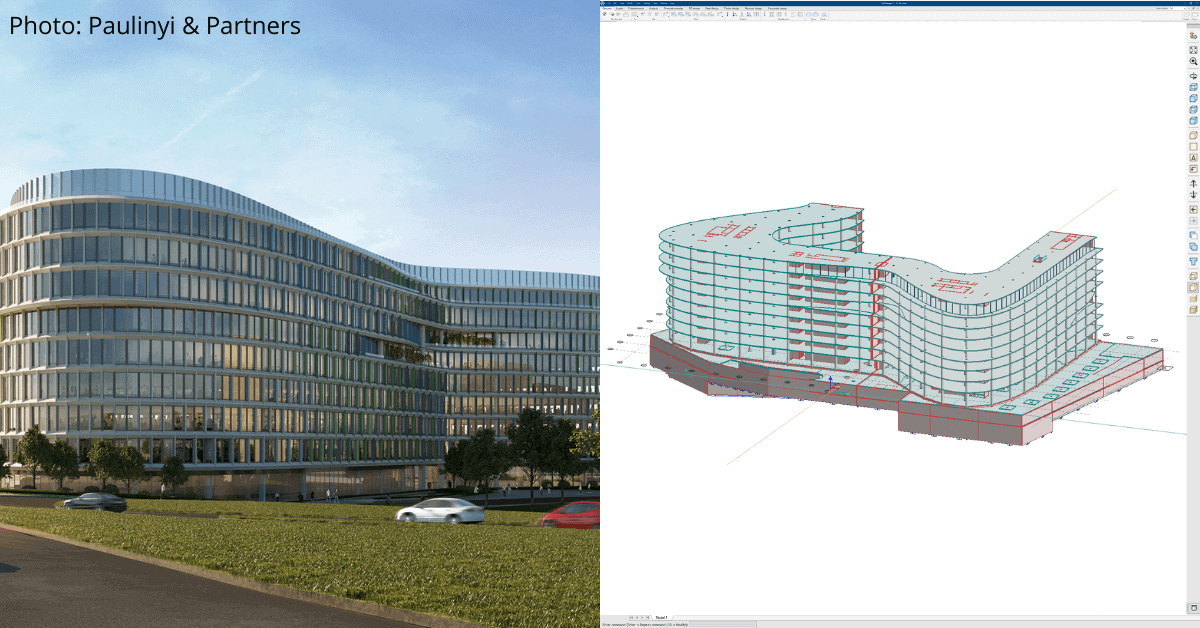
Time Saving and Precise Structural Analysis at Budapest ONE with FEM-Design
Budapest ONE is an impressive office building featuring a distinctive wavy façade and a four-leaf clover floor plan. ESIKON Ltd. selected FEM-Design as the [...]
High-Rise Structural Engineering with FEM-Design: A Feature Overview
Designing high-rise buildings presents unique challenges, requiring precise calculations, robust modelling capabilities, and the ability to anticipate the structure's behaviour in multiple scenarios. In [...]
FEM-Design 23 – Greater Efficiency: Save Time, Save Materials
FEM-Design 23 is released and introduces a brand-new 3D Bridge module, a new plastic analysis method, a renewed documentation module, and several significant improvements [...]
Bio: Mikael van de Leur is the Head of Sales at StruSoft, for the Structural Business Unit. He has been with StruSoft since 1995 and has ...show more many years hands-on experience in all our different structural software, as well as in customer relationships and sales. Mikael coordinates the Structural Sales Team and Customer experience for new customers in StruSoft’s International markets. show less
Bio: Anders Nilsen joined StruSoft in 2019 and starting of as a Sales Manager in Denmark. In 2024 Anders took on additional responsibilities as Head of ...show more Sales in Denmark, The Baltics, Hungary, Romania and Poland in the structural business unit of StruSoft. Anders has a background as a civil engineer with lots of practical experience in all our structural software. show less
Bio: Isak Björhag works as Business Development Manager for new markets. Isak has many years of experience both with hands on practical engineering and working with our ...show more customers helping them to succeed with our structural engineering software. This can be achieved through specific training, presentations or hands on demonstrations for specific cases. Please feel free to contact Isak if you are interested in a demo or want to expand your knowledge in our structural software. show less
Bio: Mohsen Ghaemi is the Regional Manager for StruSoft in our Structural Business Unit. He has a PhD in Structural Civil Engineering (Topology optimisation). Mohsen has ...show more been with StruSoft since 2002 and has more than 20 years of experience with the FEM-Design software and its development, as well as in customer relationships and sales. Please feel free to get in touch with Mohsen directly. show less
Bio: Ciprian Tibea is the Sales Manager for Baltics & Romania and has been with StruSoft since 2018. Ciprian has a PhD in Civil Engineering (Ultra ...show more High Performance Concrete Subjected to Shear Action) and a Masters Degree in Entrepreneurship and Business Administration. Prior to StruSoft, Ciprian was working as a research engineer for Consolis for 6 years and for 1 year with a local company in Romania working on structural design projects with advanced Finite Element Software (Diana and Atena). Ciprian is your main Structural Sales contact for markets outside Europe, the Baltics and Romania. Please feel free to get in touch. show less
Bio: Joni has helped and trained hundreds of engineers in structural analysis in Finland. He works in both civil and mechanical engineering fields with structural analysis. ...show more Joni has over 15 year of experience in structural design of many types and sizes of steel, concrete and timber structures. Joni is the Product Owner of our JIGI software and Country Manager for Finland. Please free to contact Joni if you need any help to solve your structural analysis problems. show less
Bio: Marek Krawczyk is the Country Manager for Poland at StruSoft. As a structural engineer, he has more than ten years of international experience, working on ...show more various projects in Europe, the UK, the USA and Asia. He joined StruSoft in 2022 to strengthen our presence in Poland and support the StruSoft software Users in the local market. show less
Bio: Jan Wróbel has over 6 years prior experience before joining StruSoft working as a structural engineer, using FEM-Design and WIN-Statik in his daily practice. His ...show more international project work has equipped him with broad technical knowledge and understanding of various design standards and client needs. He is now focusing on technical support for Polish customers. Feel free to contact Jan for any assistance with StruSoft's structural software or any other structural engineering questions! show less
Bio: Edris Mohammadi Nikzad is a Costumer Success Manager at StruSoft, based in our Stockholm’s office. He joined StruSoft in 2019 and has many years of ...show more experience on practical engineering and working with FEM-Design and WIN-Statik. Edris works closely with our customers in Sweden to help them to succeed with our structural engineering software. If you are interested in a demo of our calculation programs for a specific purpose, or if you have a special request for any training, please feel free to contact Edris. show less
Bio: Markus Mitikka is a customer coach for StruSoft in Finland. He has previous experience of FEM calculations and structural designs working for engineering office. He ...show more was a big fan of StruSoft software before joining StruSoft in beginning of 2022. Markus can help you to become more efficient with all our structural software. Please feel free to get in touch. show less
Bio: Casper Hougaard is a Customer Coach for StruSoft's Structural Team based in Denmark. He joined StruSoft in 2022 and has a background in civil engineering ...show more with lots of practical experience using FEM-Design. Casper would be happy to help any Danish FEM-Design Users with general guidance, project specific guidance and training. show less
Bio: Fredrik Lagerström MSc has 8 years prior experience before joining StruSoft working as a civil engineer using FEM-Design, WIN-Statik & PRE-Stress in his daily practice. ...show more In 2011 Fredrik joined StruSoft as a Technical Sales and Education Manager. Fredrik works closely with both Sales and Development to get feedback directly from our Swedish Customers to in turn help our Developers to implement the requested new features in an accurate way. Please feel free to get in touch with Fredrik if you require any technical help or support with our structural software. show less
Bio: Suleyman Sayed, also known as Sulo, is the branch manager for Matrix's structural engineering software products, StruSoft's sister company in The Netherlands. Currently, he primarily ...show more focuses on expanding market share and devising strategies to better position FEM-Design in the Netherlands, the Dutch Antilles, and Suriname. With his extensive background in structural engineering, deep market knowledge, and experience in various industries such as petrochemicals and civil construction, Sulo is well-equipped to offer customized solutions that perfectly meet the needs of his clients. show less
Bio: Suhas is the Managing Director of StruEngineers India, a Design & Detailing Service company 100% owned by StruSoft. Suhas has a BSc in Civil Engineering ...show more and has been providing design support services to the Precast Industry, worldwide, over the last 20 years. Suhas was one of the original founders of StruEngineers which was established 10 years ago and previously worked for Neilsoft in Pune. StruEngineers provides local support in India for all StruSoft software and Suhas is a specialist with IMPACT AutoCAD. If you are based in India and looking for more information or support please feel free to get in touch directly with Suhas. show less